What are the differences between decimal points and significant figures?

As a student studying Maths, you can't avoid discussions about decimal points and significant figures. Both play an essential role in ensuring the accuracy of calculations. But what do these terms mean, and how do they differ? 

In this guide, we'll help you understand the differences between decimal points and significant figures and provide practical tips on applying them correctly in your Maths study revision, making it an important part of any mathematical discussion.

First, what is the difference between decimal points and significant figures?

Decimal Points

These are numbers that come after the whole number and a point. They are used to indicate the fraction of a whole number. For example, in the number 3.14, the d.p. separates the 3 from the 14, indicating that it is the fraction 14/100 of the whole number 3. 

In Maths, the number of d.p. to use depends on the precision of the calculation. For instance, if you're calculating the area of a circle, you may need to use π (pi) for several decimal places.

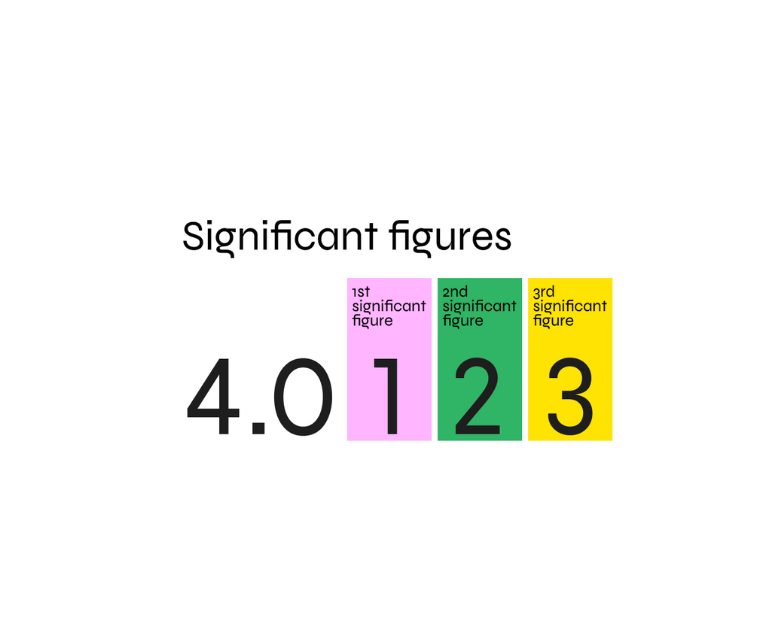
Significant Figures

Also known as significant digits, these are a way of indicating the precision of a number. They are the digits that carry meaning in a number, excluding any leading non-zero digits. For example, in 0.0032, 3 and 2 are the s.f. In scientific notation, these are shown in the coefficient (the number before the power of ten). For instance, in the number 2.36 x 10^5, the significant figures are 2, 3, and 6.

Differences Between The Two

Decimal points and significant figures differ in their purpose and the information they convey. The former indicates precision by the number of places beyond the whole number, while the latter demonstrates accuracy by counting the non-zero digits in a value. This distinction makes significant figures more suitable than decimals for expressing measurements or calculations, especially in scientific contexts.

Tips for Applying Decimal Points and Significant Figures

When working with numbers that include fractions after the point, a student needs to be mindful of both the point that separates the whole and the fractional parts, and the significant figures for accuracy. Here are some guidelines to correctly apply these concepts:

● During multiplication and division, the final answer should match the count of significant figures from the least precise measurement involved.

● For addition and subtraction, align the final result to the least precise fractional part among the measurements.

● In rounding off, if the digit following the last one to be kept is 5 or more, increase the final retained digit by 1.

Conclusion

In conclusion, decimal points and significant figures are crucial concepts in Maths that help ensure accuracy and precision in calculations. While these concepts may seem confusing at first for a student, understanding their differences and knowing how to apply them can help a student excel in Maths studies and beyond. With the tips in this guide, a student can now confidently navigate precision and effectively apply both concepts in all their Maths revision.